- #1
WMDhamnekar
MHB
- 381
- 28
Hi,
What is your probability of winning a game of tennis, starting from the even score Deuce (40-40), if your probability of winning each point is 0.3 and your opponent’s is 0.7?
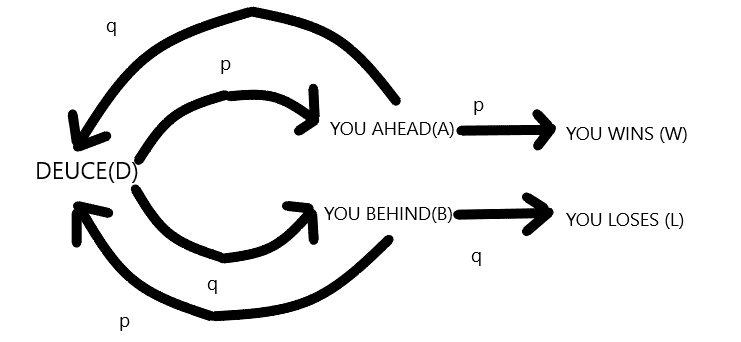
My answer:
I think the sequence of independent trials are required to win a game of tennis starting from even score Deuce(40-40),each of which is a success with probability (0.3 × 0.3 =0.09) or a failure with probability (0.7 × 0.3 + 0.7 × 0.3 =0.42). Suppose, the independent trials to win a game of tennis are n. That means after (n-1) trials of failures, the nth trial is success.
What is your probability of winning a game of tennis, starting from the even score Deuce (40-40), if your probability of winning each point is 0.3 and your opponent’s is 0.7?
My answer:
I think the sequence of independent trials are required to win a game of tennis starting from even score Deuce(40-40),each of which is a success with probability (0.3 × 0.3 =0.09) or a failure with probability (0.7 × 0.3 + 0.7 × 0.3 =0.42). Suppose, the independent trials to win a game of tennis are n. That means after (n-1) trials of failures, the nth trial is success.
Last edited: